Area And Perimeter Of Quadrilaterals

A seven min read

In Euclidean geometry, a quadrilateral is a iv-sided 2d figure whose sum of internal angles is 360°. The word quadrilateral is derived from two Latin words 'quadri' and 'latus' meaning 4 and side respectively. Therefore, identifying the properties of quadrilaterals is of import when trying to distinguish them from other polygons. Then, what are the properties of quadrilaterals? In that location are two properties of quadrilaterals:

A quadrilateral should be airtight shape with four sides
All the internal angles of a quadrilateral sum up to 360°

In this article, you will get an idea about the 5 types of quadrilaterals (Rectangle, Square, Parallelogram, Rhombus, and Trapezium) and get to know about the properties of quadrilaterals.

Hither are the v types of quadrilaterals discussed in this commodity:

Rectangle
Square
Parallelogram
Rhomb
Trapezium

Here is a video explaining the properties of quadrilaterals:

This is what you'll read in the article:

Properties of the quadrilaterals – An overview

The diagram given below shows a quadrilateral ABCD and the sum of its internal angles. All the internal angles sum up to 360°. Thus, ∠A + ∠B + ∠C + ∠D = 360°

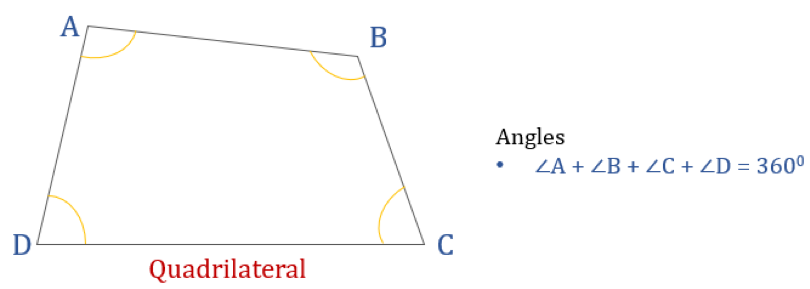
Properties of quadrilaterals and types of quadrilaterals

Properties of quadrilaterals	Rectangle	Square	Parallelogram	Rhombus	Trapezium
All Sides are equal	No	Yes	No	Yes	No
Opposite Sides are equal	Yep	Yes	Yes	Yep	No
Opposite Sides are parallel	Yes	Yes	Yep	Aye	Yes
All angles are equal	Yeah	Yep	No	No	No
Reverse angles are equal	Yes	Yes	Yeah	Yes	No
Sum of two adjacent angles is 180	Yes	Yes	Yes	Yes	No
Bisect each other	Yeah	Yes	Yep	Yes	No
Bifurcate perpendicularly	No	Yeah	No	Yeah	No

Let'south discuss each of these v quadrilaterals in item:

Here are questions which will teach y'all how to apply the properties of all v quadrilaterals you'll acquire in this article.

Rectangle

A rectangle is a quadrilateral with iv right angles. Thus, all the angles in a rectangle are equal (360°/4 = 90°). Moreover, the opposite sides of a rectangle are parallel and equal, and diagonals bisect each other.

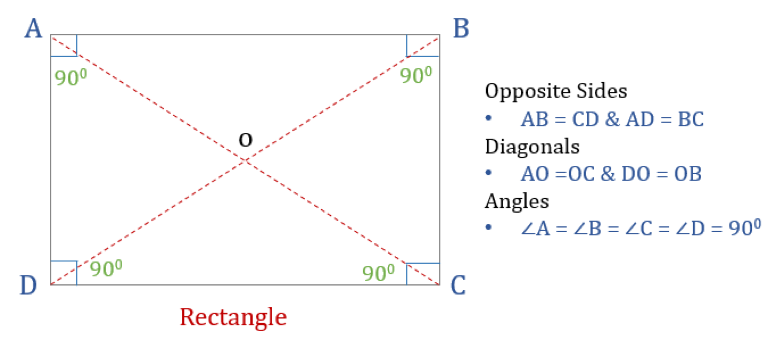
Here are the 3 properties of a rectangle:

All the angles of a rectangle are 90°
Opposite sides of a rectangle are equal and Parallel
Diagonals of a rectangle bisect each other

Rectangle formula – area and perimeter of a rectangle

If the length of the rectangle is 50 and breadth is B so,

Area of a rectangle = Length × Latitude or L × B
Perimeter of rectangle = 2 × (L + B)

These practice questions will help you solidify the backdrop of rectangles

Are you lot planning to enroll at height business schools? Let us aid yous conquer the starting time step of the procedure i.eastward., taking the GMAT. Take a gratuitous GMAT mock to empathize your baseline score and start your GMAT prep with our gratuitous trial. We are the virtually reviewed online GMAT Prep visitor with 2200+ reviews on GMATClub, every bit of fourth Oct 2021.

Did you know e-GMATers have reported more than 700+ scores than ever before in GMAT Guild'southward history? Sentinel this video to understand how e-GMAT has achieved this record-shattering result by investing and innovating with a unmarried goal in listen – To create a platform that empowers students to achieve and deliver their very best.

Foursquare

Foursquare is a quadrilateral with four equal sides and angles. It's besides a regular quadrilateral as both its sides and angles are equal. Just like a rectangle, a square has four angles of 90° each. It tin as well be seen as a rectangle whose two adjacent sides are equal.

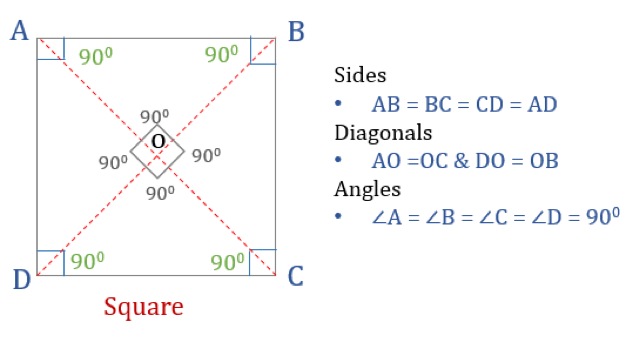
Here are the three backdrop of a Foursquare:

All the angles of a square are 90°
All sides of a foursquare are equal and parallel to each other
Diagonals bisect each other perpendicularly

Square formula – area and perimeter of a square

If the side of a square is 'a' then,

Area of the square = a × a = a²
Perimeter of the square = 2 × (a + a) = 4a

These practice questions will help you solidify the properties of squares

Scoring a Q50-51 on the GMAT helps you lot get a 700+ GMAT score. Start your journey of getting a Q50-51 on the GMAT with e-GMAT's AI-driven online preparation course. Our xPERT non just curates the most optimized learning path merely as well tracks your comeback, ensuring that you get to your target Quant score quickly and reliably. Watch this video to know more:

Parallelogram

A parallelogram, as the proper noun suggests, is a elementary quadrilateral whose opposite sides are parallel. Thus, it has two pairs of parallel sides. Moreover, the opposite angles in a parallelogram are equal and their diagonals bisect each other.

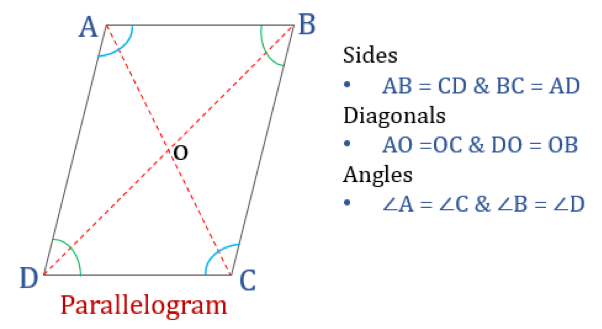
Hither are the 4 properties of a Parallelogram:

Opposite angles are equal
Contrary sides are equal and parallel
Diagonals bisect each other
Sum of any two side by side angles is 180°

Parallelogram formulas – area and perimeter of a parallelogram

If the length of a parallelogram is '50', breadth is 'b' and tiptop is 'h' then:

Perimeter of parallelogram= 2 × (l + b)
Surface area of the parallelogram = l × h

These exercise questions will assistance you lot solidify the properties of parallelogram

Rhomb

A rhombus is a quadrilateral whose all four sides are equal in length and opposite sides are parallel to each other. However, the angles are non equal to 90°. A rhombus with right angles would go a square. Another name for rhombus is 'diamond' as it looks similar to the diamond suit in playing cards.

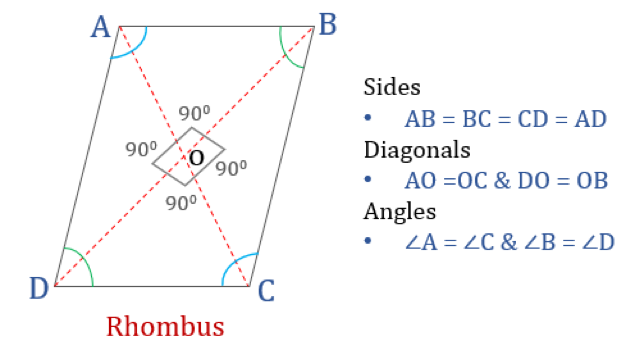
Here are the four properties of a Rhomb:

Contrary angles are equal
All sides are equal and, opposite sides are parallel to each other
Diagonals bifurcate each other perpendicularly
Sum of any ii adjacent angles is 180°

Rhombus formulas – surface area and perimeter of a rhomb

If the side of a rhombus is a then, perimeter of a rhomb = 4a

If the length of two diagonals of the rhombus is d_i and d₂ then the area of a rhombus = ½ × d₁ × d_ii

These practice questions volition help y'all solidify the backdrop of rhombus

Trapezium

A trapezium (called Trapezoid in the U.s.) is a quadrilateral that has only ane pair of parallel sides. The parallel sides are referred to every bit 'bases' and the other 2 sides are called 'legs' or lateral sides.

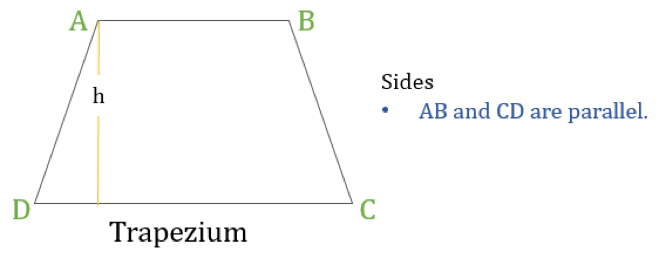
A trapezium is a quadrilateral in which the following 1 property:

Only 1 pair of opposite sides are parallel to each other

Trapezium formulas – area and perimeter of a trapezium

If the height of a trapezium is 'h'(every bit shown in the above diagram) then:

Perimeter of the trapezium= Sum of lengths of all the sides = AB + BC + CD + DA
Area of the trapezium = ½ × (Sum of lengths of parallel sides) × h = ½ × (AB + CD) × h

These exercise questions will help you solidify the properties of trapezium

Properties of Quadrilaterals – Summary

The below image as well summarizes the properties of quadrilaterals

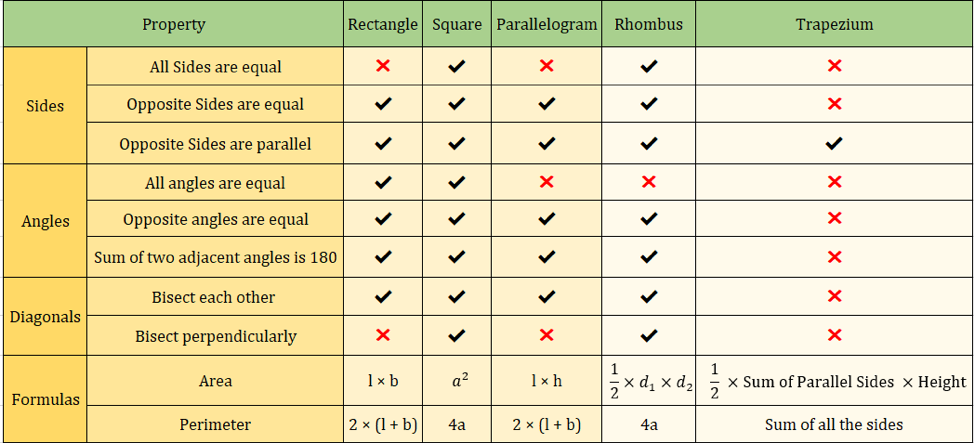
Of import quadrilateral formulas

The below table summarizes the formulas on the expanse and perimeter of unlike types of quadrilaterals:

Quadrilateral formulas	Rectangle	Square	Parallelogram	Rhomb	Trapezium
Expanse	50 × b	a²	l × h	½ × d1 × d2	½ × (Sum of parallel sides) × height
Perimeter	2 × (l + b)	4a	two × (l + b)	4a	Sum of all the sides

Further reading:

Circle Formulas – Expanse and Perimeter
Properties of Numbers – Even & Odd | Prime | HCF & LCM
Properties of Triangles – Definition | Types | Classification
Lines and Angles – Properties and their Application

To ace the GMAT a well-defined study plan is required. Save threescore+ hours on GMAT preparation by post-obit these three steps:

Quadrilateral Do Question

Let's practice the application of properties of quadrilaterals on the post-obit sample questions:

GMAT Quadrilaterials Do Question 1

Adam wants to build a fence around his rectangular garden of length x meters and width 15 meters. How many meters of debate he should buy to contend the entire garden?

xx meters
25 meters
30 meters
xl meters
l meters

Solution

Pace i: Given

Adam has a rectangular garden.
- It has a length of 10 meters and a width of 15 meters.
- He wants to build a fence around it.

Step two: To find

The length required to build the fence around the entire garden.

Pace 3: Approach and Working out

The fence can simply exist built around the outside sides of the garden.

And so, the total length of the contend required= Sum of lengths of all the sides of the garden.
- Since the garden is rectangular, the sum of the length of all the sides is goose egg but the perimeter of the garden.
- Perimeter = 2 × (10 + 15) = fifty metres

Hence, the required length of the contend is l meters.

Therefore, option East is the right answer.

GMAT Quadrilaterials Practice Question 2

Steve wants to paint 1 rectangular-shaped wall of his room. The price to paint the wall is $1.5 per square meter. If the wall is 25 meters long and 18 meters wide, then what is the full cost to paint the wall?

$ 300
$ 350
$ 450
$ 600
$ 675

Solution

Step ane: Given

Steve wants to pigment one wall of his room.
- The wall is 25 meters long and eighteen meters wide.
- Cost to paint the wall is $1.v per square meter.

Stride 2: To find

The full cost to paint the wall.

Step 3: Arroyo and Working out

A wall is painted across its entire area.
- So, if we find the total surface area of the wall in square meters and multiply it past the cost to paint ane foursquare meter of the wall then we can the total cost.
- Area of the wall = length × Breadth = 25 metres × eighteen metres = 450 square metre
- Total cost to pigment the wall = 450 × $1.5 = $675

Hence, the right answer is option E.

We promise by now you would have learned the dissimilar types of quadrilaterals, their properties, and formulas and how to apply these concepts to solve questions on quadrilaterals. The application of quadrilaterals is important to solve geometry questions on the GMAT. If you are planning to take the GMAT, we can help you with high-quality written report textile which you can access for gratuitous by registering hither.

Here are a few more articles on Math:

Improve accurateness in Math questions on Polygons
Geometry Questions – Nearly Common Mistakes | GMAT Quant Prep

Watch this GMAT geometry-complimentary webinar where we talk over how to solve 700-level Information sufficiency and Trouble questions in GMAT Quadrilaterals:

If you are planning to take the GMAT, nosotros can give you access to quality online content to prepare. We are the most reviewed GMAT prep company on gmatclub with more than than 2200+ reviews, as of 4th Oct 2021.

Write to united states at acethegmat@e-gmat.com in instance of whatever query.

FAQs

What are the different types of quadrilaterals?

There are 5 types of quadrilaterals – Rectangle, Square, Parallelogram, Trapezium or Trapezoid, and Rhombus.

Where tin I find a few do questions on quadrilaterals?

Y'all can detect a few practice questions on quadrilaterals in this article.

What is the sum of the interior angles of a quadrilateral?

The sum of interior angles of a quadrilateral is 360°.